The Nature of Solutions to System of Linear Equations
Nature of Solutions for System of Two Linear Equations
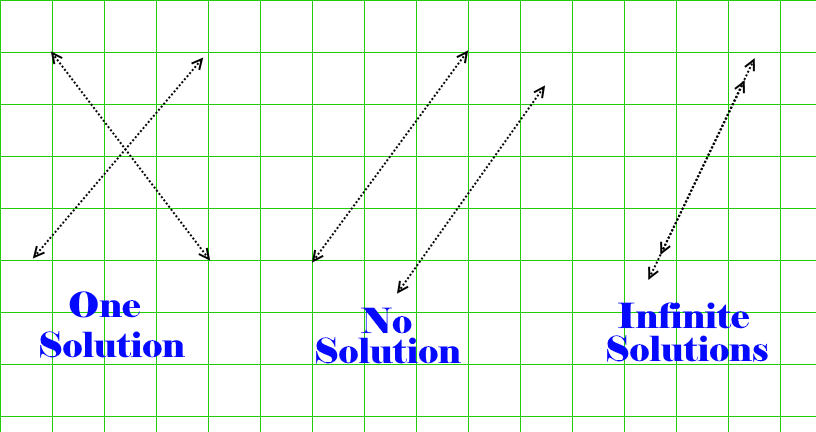
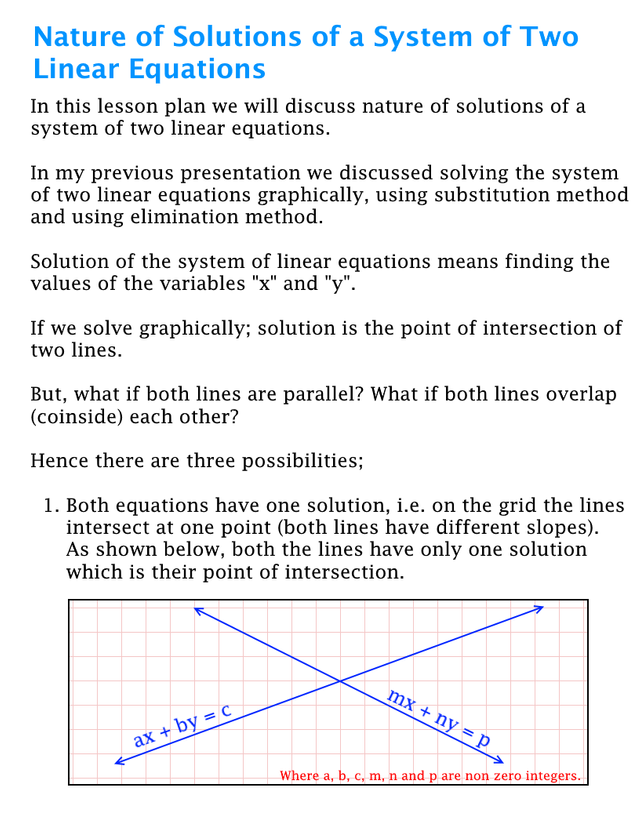
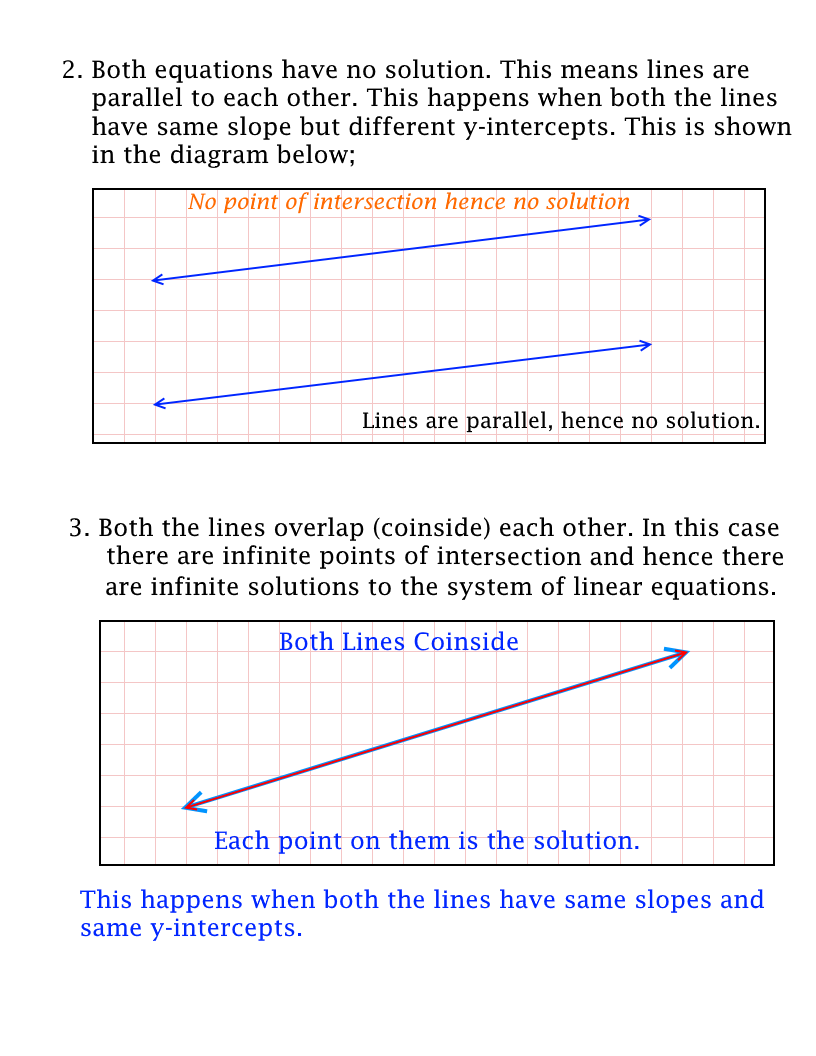
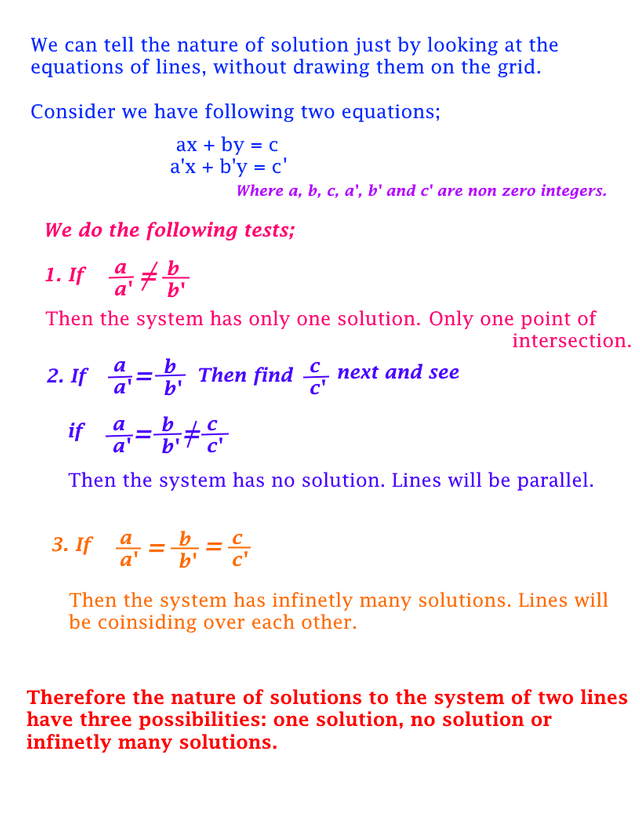
This is the warp up for the concept of solving system of two linear equations




This is the warp up for the concept of solving system of two linear equations
Thanks a lot @steemiteducation. I really appreciate your support.
I really enjoy these posts as they refresh my memory on these equations and such that I used to know by heart. I think I was possibly one of the very few in my classes over the years that truly enjoyed math.
Keep up the good work @mathworksheets!
Thanks a lot for you feedback my dear friend @liberty-minded. I think it's a blessing to be excellent in math or being a math lover.
The University of Steem! Well done!
Thanks for the appreciation dear brother.
helpful stuff.. lovely work my friend
cheers!
Thanks for the support my dear friend @dinsha.
Better apply now for NASA @mathworksheets I'm telli'n ya.
@cryptopie, NASA is a broke bunch of scammers, he doesn't belong there. And we need smart people like @mathworksheets here on Steemit to create a decentralized World :)
Thanks for your kind words my dear friend @cryptopie. I think NASA will scare away from my beard!LOL.
Very clear to understand.
Thanks for the appreciation dear brother.
Can't you solve the two equations simultaneously?
Thanks for the input dear friend @outwalking. This post is about telling the nature of solution without solving the equations. Previously I did posts on how to solve these equations.
Oh sorry I didn't see your other post. Sorry.
It's all right dear friend @outwalking. Actually I should leave links to my previous posts. Next time I will make sure to link same concept posts.
Thank you for your time. Have a good evening.
Ok, so is it possible for two lines to be exactly superposed on each other, so that their yield an infinite number of solutions? How can we make two parallell lines, without any solutions, to superpose as to yield an infinite number of solutions? Maybe I am just confused... Anyway, Whale done:)!
Ha haa! Whales don't ask questions!!