Parametric Calculus: Tangents
In this video I go over how calculus can be used when dealing with parametric equations. Some of the concepts that we can use calculus for on parametric curves is to determine tangents, areas, arc length, and surface area. In this particular video I take a look at determining tangents, or the slope of a point on a parametric curve in the x-y graph. Some sets of parametric equations can be written in the form of y = F(x) by first eliminating the parameter. For these particular types of functions, I show that the slope at any point can be determined by the ratio of the slopes of the y and x variable, but in terms of the parameter. If the parameter is t then we have dy/dx = (dy/dt)/(dx/dt). This means that we can determine the slope without having to eliminate the parameter! This is very useful for sketching parametric curves, which I will show in my later video so stay tuned. This is an extremely useful video to understand how it is possible to determine the slope of a line tangent to a parametric curve, so make sure to watch this video!
Watch on VidMe: https://vid.me/HXlUF
Watch on BitChute: https://www.bitchute.com/video/xNu9TaUh5ozT/
Download PDF Notes: https://1drv.ms/b/s!As32ynv0LoaIhuIiMz7ewL8u-T6sQA
View Video Notes Below!
Download These Notes: Link is in Video Description.
View These Notes as an Article: https://steemit.com/@mes
Subscribe via Email: http://mes.fm/subscribe
Donate! :) https://mes.fm/donate
Reuse of My Videos:
- Feel free to make use of / re-upload / monetize my videos as long as you provide a link to the original video.
Fight Back Against Censorship:
- Bookmark sites/channels/accounts and check periodically
- Remember to always archive website pages in case they get deleted/changed.
Join my private Discord Chat Room: https://mes.fm/chatroom
Check out my Reddit and Voat Math Forums:
Buy "Where Did The Towers Go?" by Dr. Judy Wood: https://mes.fm/judywoodbook
Follow My #FreeEnergy Video Series: https://mes.fm/freeenergy-playlist
USEFUL TIP: If I'm going too fast or too slow, play this video at a different speed.
-- TOP SECRET LIFE HACK: Your brain gets used to faster speed (#Try2xSpeed)
-- #Try4xSpeed by Replacing youtube.com with hooktube.com in the video URL ;)
Calculus with Parametric Curves
Having seen how to represent curves by parametric equations, we now apply the methods of calculus to these parametric curves.
In particular, we solve problems involving tangents, area, arc length, and surface area.
Tangents
In my earlier videos, we saw that some curves defined by the parametric equations x = f(t) and y = g(t) can also be expressed, by eliminating the parameter, in the form y = F(x).
- I will go over the General Condition under which this is possible, in a later video, so stay tuned!
If we substitute x = f(t) and y = g(t) in the equation y = F(x), we get:
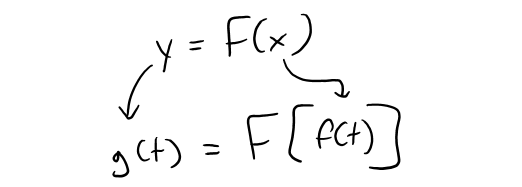
Thus if g, F, and f are differentiable, the Chain Rule gives:
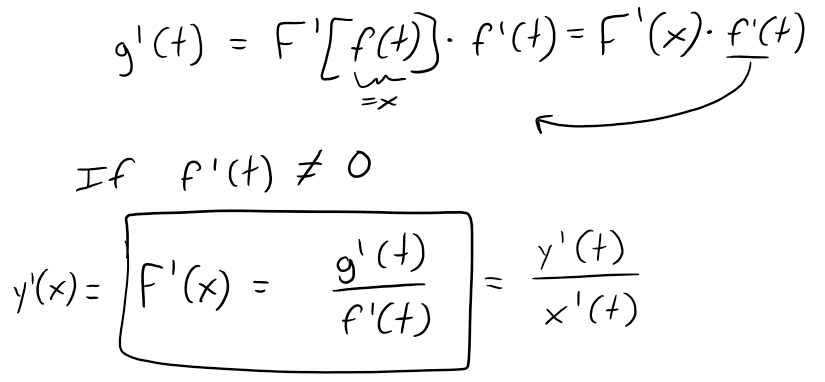
Since the slope of the tangent to the curve y = F(x) at (x, F(x)) is y' = F'(x), the equation above enables us to find tangents to parametric curves without having to eliminate the parameter!
Using Leibniz notation, we can rewrite the above equation in an easily remembered form:
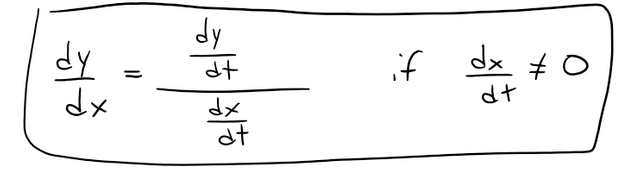
Note: If we think of a parametric curve as being traced out by a moving particle, then dy/dt and dx/dt are the vertical and horizontal velocities of the particle and the above formula says that the slope of the tangent is the ratio of these velocities.
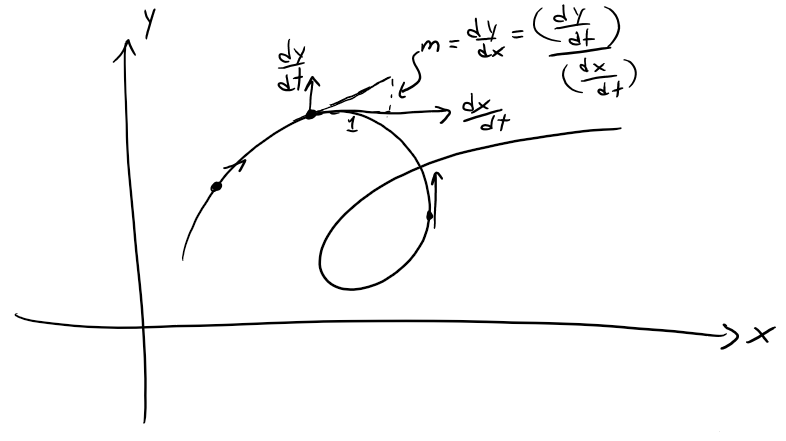
It can be seen from the above equation that the curve has a horizontal tangent when dy/dt = 0 (provided that dx/dt ≠ 0) and has a vertical tangent when dx/dt = 0 (provided that dy/dt ≠ 0).
This information is useful for sketching parametric curves.
As we know from earlier videos on Curve Sketching, it is also useful to consider d2y/dx2.
This can be found by replacing y by dy/dx in the above equation:
