Bitcoin Mining Strikes a Rich Vein of Reality

Welcome to the sixth post in a series about bitcoin mining basics. Last time, I introduced the concept and basic methodology of discounted cash flows for estimating the value of a proposed mining operation. Now I'll build on that foundation and refine the calculations for more realistic results.
What's the Real Discount Rate?
In the last post, I used a discount rate of 50% for example calculations, emphasizing that the figure was chosen for demonstration purposes only. So what is the right rate for bitcoin mining? The simple answer is 'a couple hundred percent.' You read that correctly.
Investment analysts usually build the discount rate by starting with a 'risk-free rate' (such as the US Treasury Bill yield), then adding factors corresponding to the volatility and expected growth of the subject security. The resulting discount rates typically fall within a few points of 10%. In other circumstances, investors might choose a discount rate based on what they demand of an investment in order to justify committing to it. Again, this usually generates discount rates of 10 to 20%. Higher rates, perhaps around 30%, might apply when deciding whether to buy an existing small business or invest in a start-up company. Higher rates reflect the higher risk of such investments.
Bitcoin mining falls into an entirely different regime of discount rates, for one reason: mining difficulty.
Here Comes the Math Again!
The present value of a single payment S, n periods (days, weeks, months, etc) in the future is
PV = S/(1+r)^n,
where r is the discount rate for one period.
Let's review the expected bitcoin revenue from an earlier post in this series:
R = B H / k D ,
where R = daily gross revenue in BTC,
B = 12.5 BTC = the block reward,
H = the mine's hashrate in TH/s,
D = the current mining difficulty in trillions, and
k = 49,710 hash/s, a constant from the bitcoin blockchain.

Today, the mining difficulty is 3.007 trillion. Since November 2017, the difficulty has increased at a rate of about 10% every two weeks. So if D is today's difficulty, we can guess that in two weeks, daily revenue will drop to about
R(in two weeks) = B H / k D / (1 + 10%) = R(today) / (1 + 10%).
In four weeks, the difficulty will probably increase another 10%, so
R(in four weeks) = R(today) / (1 + 10%)^2.
Looks familiar? The increasing difficulty has exactly the same effect as discounting at a fortnightly rate of 10%. That's an annual rate of 260%!
In the last post, we considered the example of an ASIC miner running at 14 TH/s drawing 1.4kW of electricity at $0.12/kWhr. Initially, the example machine produced 0.0012245 BTC/day at a cost of 0.0004686 BTC/day for power, for a net daily income (excluding depreciation) of 0.0007559 BTC. As shown below, the projected difficulty increases take away about 70% of the mine’s value compared to the original sample calculation.
Present Value of 0.0007559 BTC/day for two years:
| Discount Rate | Present Value |
|---|---|
| 50% | 0.3487 BTC |
| 260% | 0.1055 BTC |
What About Expenses?
So far, I have applied the discounted cash flow method to the bitcoin revenue stream after deducting operating expenses, using a single discount rate. That's a valid approach if revenues and expenses always rise and fall in lockstep, which they don't. Therefore, we should break up the analysis to allow different treatments for revenue and expenses. Using U to represent the daily utility expenses in dollars and E for the exchange rate,
PV(mine) = PV( BH/kD ) - PV( U/E ) .
Expressing it this way shows that the exchange rate acts on expenses exactly the same way difficulty acts on revenues. Unlike the difficulty, the bitcoin price has not enjoyed a 260% growth rate.
Also unlike the difficulty, the exchange rate defies modeling with a simple discounting formula. In fact, it defies any attempt to model it. Until something better comes along, I think it's reasonable to use an average expected bitcoin price, and not discount the operating expenses at all.
Six Months to Live
With rapidly increasing mining difficulty driving gross revenue into the ground, we have to consider the impact on a mine's productive life. The estimated daily gross revenue after the nth difficulty increase is
R(n) = R(0)/(1+r)^n,
where R(0) represents the daily revenue when mining begins. Using an undiscounted daily operating cost C, mining becomes unprofitable (reaches electrical breakeven) when
C > R(n), or
(1+r)^n > R(0)/C .
Taking the logarithm of both sides yields
n > log( R(0)/C ) / log(1+r) .
Using the same figures for a 14 TH/s ASIC miner, and difficulty increasing 10% every two weeks,
n = log( 0.0012245/0.0004686 ) / log(1.1) = 10.07 .
Once the difficulty has increased 10% ten times (about twenty weeks), the example machine reaches electrical breakeven. With the eleventh difficulty increase, bitcoin becomes less expensive than the electricity to mine it, and mining must cease. The operating expense figure above was based on bitcoin at $8,600. If the exchange rate doubles, halving the operating expense, the mine's profitable life increases to 17 cycles (34 weeks). Simply stated, if this difficulty trend continues, this strawmine has about six months to live.
Value of the Short-Lived Mine
Finally, let’s combine these ideas for the hypothetical 14 TH/s ASIC miner. We want the present value of the gross revenue stream (initially 0.0012245 BTC/day), discounted at 10% biweekly for twenty weeks, reduced by the average daily electricity expense (0.0004686 BTC/day), undiscounted. Note this is a total of eleven two week periods.
PV(mine) = PV(10%, 11, 14 * 0.0012245) – 11 * 14 * 0.0004686
= 0.05031 BTC
The table below shows the gross revenue, utility expense, and net income for each two-week period until the system falls below electrical breakeven.

Method Madness
The next table summarizes the results of the methods discussed in this series.
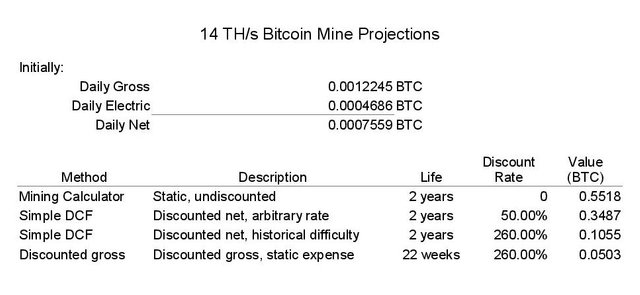
The right-hand column shows each method's estimate of value for the same mining operation. Each figure represents a calculation of how much bitcoin the mine would give you if it returned all of it immediately, rather than gradually over its life. If setting up the mine costs more than that, you would do better to buy that much bitcoin instead.
Each row represents an attempt to build more reality into the model. Each tries to predict the future, and none is any better than the assumptions behind it. The first row assumes the mine produces bitcoin at the same rate from beginning to end, a naive and sentimental approach at best. There is no rational reason to believe in constant mining returns, and plenty of reason not to believe in them.
The second row acknowledges that things might change over time, and that uncertainty means payment delayed equals payment devalued. It fails, however, in not having a factual basis for the devaluation (the discount rate). Without an independent means of setting a model's parameters, users too easily fall into the trap of choosing parameters to produce a desired result.
The third row differs from the second only in making a reasoned, logical choice of the discount rate. The dominant factor driving mining output, the difficulty, has a history of rapid growth, with overwhelming impact on mining profits. This method has the flaw of applying the same enormous discount rate to both revenue and expenses, effectively assuming that the exchange rate rises along with the difficulty. As a consequence, the model mathematically eliminates the risk of the mine falling below electrical breakeven. It also carries the assumption that the difficulty (and price!) will continue for two more years to grow at its present rate.
The final row corrects the error of strongly discounted operating expenses. This allows the model to predict a loss-driven shutdown, which does happen with obsolete equipment in the real world. The model retains the assumption of continued rapid growth in mining difficulty, although the shutdown mechanism shortens the time horizon to six months.
Along with its shortcomings, each model allows flexibility in adjusting the underlying assumptions and exploring 'what if' scenarios. The more you explore, the more insight you gain into what changes affect the outcome under what circumstances, and by how much. The goal is to use that insight to make better, or at least better reasoned, decisions. Thanks for reading!
Other posts in this series:
Bitcoin Mining Profitability Perspective - 1 Feb 2018
So You Want to Start a Bitcoin Mine - 3 Feb 2018
Operating a Bitcoin Mine - 7 Feb 2018
How Much Does Mined Bitcoin Cost? - 11 Feb 2018
Time-Travel Voodoo for Mining Decisions - 15 Feb 2018
Next Post: To Be Determined
Congratulations @mountainherb! You received a personal award!
Click here to view your Board
Congratulations @mountainherb! You received a personal award!
You can view your badges on your Steem Board and compare to others on the Steem Ranking
Vote for @Steemitboard as a witness to get one more award and increased upvotes!