The Mathematics of Fractional Reserve Banking
Tired of the Crookedness of the Financial System?
Q: What's the REAL underlying mechanism of Fractional Reserve Banking?
A: Preying on people's inability (or willful ignorance) to understand mathematics!

Once upon a time, Greek philosophers posed many problems which were of great interest in the day. Mathematicians now understand the literal interpretation of these questions as certain types of calculus problems. Modern-day philosophers, on the other hand, interpret each of these so-called paradoxes in metaphysical notions.
For our purposes, we will focus on the mathematical aspects of these philosophical questions, as they relate directly to the implementation of Fractional Reserve Banking.

Zeno's Paradox
A man is walking towards a destination and only moves halfway between his current location and where he's going. As such, the man must stop an infinite number of times before he proceeds to the next halfway point and, as such, will never attain his goal.
However, the distance between a man and his destination is finite. Surely, he will make it to his destination!
In fact, he does reach his goal in what is known as the limit or at infinity.
The Limit of Zeno's Paradox
Limits are notions that describe behavior in an aysmptotic sense, that is to say, in a general sense as some varying parameter approaches a particular value. The general rule is that one does not care what is happening precisely at the point that is being approached but rather is interested in the overall pattern in all possible paths that approach the destination.
I won't discuss the mathematics of limits (as one can abstract the notion further, especially when dealing with topological spaces), but the above description gives a decent picture. To reiterate: when limits are used, we do not care about the final limiting value, only in the qualitative behavior of all possible paths that approach the point of interest.
In the example where a man moves halfway from his current location to his destination, we can describe the infinite sum of all the finite distances that he moves in approaching his goal.
Infinite Sequences and Sums
(Since MathJax is not implemented, bear with me on not having decent mathematical notation.)
We describe this infinite sum by first considering the (finite) partial sum of all the first k steps.
First, we need to define the distance between the starting point and the end point to be some arbitrary value, a.
The distance that the man initially moves is a/2. The second distance is a/4, the third a/8, and so on. In fact, the distance of the k'th step of the man is a/(2^k).
Let us consider the sum of the first k distances. The first sum is a/2, the second sum 3a/4, the third sum 7a/8, and so on.
We write this as SUM(i=1..k, a/2^i) = S_k = (2^{k}-1)a/(2^k) .
If we take the limit of k as k approaches infinity, we see that the factor (2^{k}-1)/2^k approaches 1. In other words, if we say that M is a very large number, then M - 1 is also very large, and the ratio of (M - 1) / M becomes closer to 1 as M increases. Indeed, this is precisely the case when M = 2^k.
So you see, in the limit, the sum of the total distance traveled approaches a, which intuitively makes sense as that is the distance we defined between the start point and the end point.
A Picture is worth a 1000 words
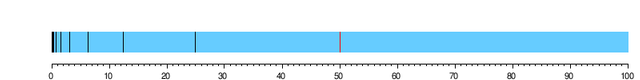
Here, 100 represents 100% of a and starting on the right, we move halfway towards 0. Near 0, we have an accumulation of points near the value we are approaching. In mathematics, we also say that a limit point is an accumulation point.
Infinite Geometric Series
The above example is nothing more than an application of an infinite geometric series.
An infinite geometric series is an infinite series of numbers where each value in the series is given by a (finite) partial sum. A partial sum is the sum of a finite set of numbers in a sequence. More generally, we write that the k'th finite value in an infinite sequence is given by
a_k = a r^{k},
where r is some geometric ratio that defines the change between one number in the sequence to the next.
The partial sum of the a_k's is given by s_k = SUM( i=0..k, a_k ) = a (1-r^k)/ (1-r).
The a_k's form a sequence and the s_k's form a series.
Without going into the details, s_k converges to a finite value as k approaches to infinity under the condition that |r| < 1.
This infinite sum converges to the value a / (1 - r).
Wutz Dis Gotta Due Wit' Moneez?
The mathematics of any monetary system that uses a fractional reserve policy is based entirely on infinite geometric series.
Ever heard of the money multiplier effect?
The lower the on-hand reserve requirement, the larger the money multiplier effect. Suppose a bank has a reserve requirement as low as 2.5%. Then, this equates to 39x increase in the monetary supply by doing nothing more than lending.

We first need to understand how a fractional reserve system operates. Then, we will see if we can spot the pattern of an infinite geometric series.
Ignoring all the intricacies of how the U.S. Treasury issues bonds in exchange for Federal Reserve Notes, suffice to say that someone (Congress) is doling out money to someone. So what's happening?
Say you are on the receiving end of Congress (although, normally, when I think of that phrase, I don't think of good things), and you are a contractor and manage to obtain a bid from the government to build a new bridge in the desert. Let's call your business, Business 0, and your bank, Bank 0.
You are appropriated x amount of Federal Reserve Notes. You take these notes and deposit them into Bank 0. Bank 0, being in the business of money lending, loans your money to a new business, Business 1, keeping only the minimum reserve requirement on hand. Business 1 receives the loan and places the money in Bank 1. Bank 1 creates a loan for Business 2 which in turn deposits the money in Bank 2. This process repeats ad infinitum.
What we are going to do is consider two different pairs of columns. One pair will be the two columns that contain the total sum that is loaned out by all the previous banks and the amount loaned out by the current bank. The other pair of columns will contain the sum of all the reserve money kept in all the previous banks and the reserve money kept in the current bank.
For simplicity, we will say that there is a reserve requirement of 10% and that the amount of money awarded in the contract bid was only $100,000,000. That is to say if we go back to our partial sums and infinite geometric series, we have that a = 10,000,000 and 90,000,000 for the Money Lent and Money in Reserve columns, respectively, and that and r = .9.
| Bank Number | Sum of Money Lent | Money Lent | Money in Reserve | Sum of Money in Reserve |
|---|---|---|---|---|
| 0 | 90,000,000 | 90,000,000 | 10,000,000 | 10,000,000 |
| 1 | 171,000,000 | 81,000,000 | 9,000,000 | 19,000,000 |
| 2 | 243,900,000 | 72,900,000 | 8,100,000 | 27,100,000 |
| 3 | 309,510,000 | 65,610,000 | 7,290,000 | 34,390,000 |
| 4 | 368,559,000 | 59,049,000 | 6,561,000 | 40,951,000 |
| ... | ... | ... | ... | ... |
| k | 90,000,000*(1-.9^k)/(.1) | 90,000,000*(.9)^{k} | 10,000,000*(.9)^{k} | 10,000,000*(1-.9^k)/(.1) |
You see that the columns correspond to a two different pairs of geometric sequences and series.
Observe that the total amount of money held in reserve by all the banks as we approach infinity (and note that only after 4 iterations, we are almost halfway there) is the initial amount for the original contract, i.e. $100,000,000. But, wait, there's all that money that is loaned out to people now. There is $900,000,000 of that funny money floating around for only $100,000,000 kept in the banks.
The money multiplier effect can be calculated by taking (1 / reserve requirement %) - 1. For banks, who have a reserve requirement of 10%, we see that the money multiplier effect is 9 times.
Fun fact: Canada, the UK, New Zealand, Australia, and Sweden have no reserve requirement!
This is how a fractional reserve banking system creates money from nothing. Notice that we didn't even have to use usury (charging interest on loans!) to create new currency.

Hey @complexring, I mentioned your blog on my latest post. https://steemit.com/learntocode/@veryscience/learn-how-to-code-how-to-create-your-first-webpage-from-start-to-finish-with-html-and-css
This is fascinating!
I did not realize that fractional reserve banking could be modeled as an infinite geometric series, but it makes sense.
One Question -- "Sum of Money Lent" when k (bank number) = 4...
Shouldn't that be 368,559,000 rather than 315,414,900?
I was following along with you, and got the same numbers up until that point.
Just wondering if I went wrong somewhere.
Thanks for this eye-opening article!
What would I do without you fixing all of my math mistakes?
I'm not at your level in math, but I'm smart enough to know I can learn from you if I read your posts carefully and work through the examples you present. Thanks for taking the time to produce quality technical articles!
Who says you can't incentivize quality teachers?
scam.
This is the worst informed thread I've ever seen that's not on Facebook. The post is fine, the comments are ridiculously awful.
Are all the comments ridiculously awful, including this one (and yours)?
It is a comment isn't it? I see no reason why any comment depending on the persons perspective couldn't be viewed as ridiculously awful. It's all subjective anyways when making distinctions.
Come on, don't compare us to Facebook! I thought we were better than this ;-)
I always appreciate original research, or just a new look on things. I even understand quite a bit of math myself. But this post is complete bullshit, there's absolutely zero connection between limits and fractional reserves. Sure, you can apply any formula to any phenomenon with enough substitutions, but that doesn't imply a meaningful relationship.
The fact that this post is scoring so well, can be both seen as a general inability to understand mathematical principles by normal people (which I don't mind at all) or as a "riding-the-whale" circlejerk of upvotes (which I am finding more and more annoying).
I disapprove of people misunderstanding mathematics.
I also disapprove of my complete lack of misunderstanding of economics.
Explain to me why you approve of the general population not understanding mathematics?
Or maybe I didn't understand your comment properly ... probably so. I am almost always incorrect.
I don't mind the general population not understanding advanced mathematics. Just as I expect people to don't mind I don't speak French fluently.
Infinite geometric series and sequences are not necessarily all that advanced. We've been studying, constructing, and observing such objects for at least 2 millennia and are relatively basic.
Neither is speaking French, if you speak French.
And what's so bad about trying to teach people the language that I happen to be the most fluent in?
Trying to teach anything is a noble cause and can only be encouraged. But it's important to understand not everybody needs French, and plenty of people dislike French enough to TL;DR anything related. It's this last option I think is the case for many upvoters of this post. And I know I sound jealous (I am, a bit) but I dislike the riding-the-whale voting on this platform.
I'm trying to create a math blog, and I just happened to explore a topic that both interests me as well as the general audience (many blockchain enthusiasts). Most people here dislike the banking system (hence, why they are cryptotoken users).
The purpose of this post was to illustrate money multiplication via reserve rates and demonstrate how infinite geometric series can be applied to my understanding of the system at that time.
My illustration may have been a poor example to what happens in the real world, but whether banks borrow from the central bank in order to meet RR before a loan is made or after a loan is made does not change the underlying principle of how money multiplication, via deposits in a bank.
So, yes, one could have instead simply used the (inverse of 1 - RR) - 1 and expressed this as the maximal money multiplier that could have occurred and stated that the actual MM is determined by numerous factors, including a ratio of how much of a particular loan is kept in cash, not used to pay off other businesses, and is therefore not deposited into a new bank account. And in fact, you'll note that I do state that is the maximal MM that can exist and indeed derive it from using the infinite geometric series model. Coincidence?
I could do a completely new post that explains this in light of all the new economics that I have learned from @sigmajin and yourself, if you think it would prove worthwhile.
I don't have the feeling I have anything I can learn you, tbh :)
As for the new article, please no, forvtwo reasons: 1) I don't want to be the cause, even partly, for anyone doing anything and 2) after this discussion, I think the OP+thread is a very complete assessment of the concept.
And maybe a 3) I'd much rather see you work on another blogpost, next in the series...
Meanwhile, check out my latest post I linked here somewhere. That'll give you an idea how bad I am at economics, lol. And there are some questions I'd like to see answered by more capable people...
Also, if you want to write an article (or 3) on the myths of the money multiplier effect, I'm all for learning more ...
I also think that when someone just comes in and says you are wrong and leaves it at that and doesn't explain your failings, you do both him and yourself a a great disservice.
Ok, let's try this: why do you need limits to describe something that can be expressed as a simple percentage?
Because it is a deeper analytical method of mathematics that can be used to understand the subject and in fact you need limits to really understand how an infinite geometric series really operates.
Yeah, the "loaners/sellers" wasn't meant as a quote or anything, it's just the simplified concepts my brain uses to try understand all this :)
Ok, I get your point. I'm not nearly as good in math as you, and much less in translating math to English. I was hoping to do what you suggested and write one (3?) articles about this. However, I can't get it working in my head... See this post, feedback highly appreciated. Same for our friend @sigmajin
https://steemit.com/steemit/@r4fken/fractional-reserve-banking-and-steemit-questions-for-experts
Yes, you need limits for infinite series. Not for percentages. It feels like a farfetched look-how-smart-i-am post.
Also, the assumption that loaners pay and sellers just deposit again is flawed.
I don't see how percentages would get point across of money multiplication as well as how this model (albeit, imperfectly) fits with an infinite geometric series.
This is an example I've used in my Calculus II courses numerous times when introducing infinite geometric series and sequence.
Can you provide an example of how percentages describe this phenomena?
In response to the loaners and sellers comment ... I never used the terminology of sellers and loaners, so it looks like you're interpreting that I said certain statements that I did not?
Maybe you could provide a quote or an example with some more meat on the bone to back up what you are claiming that I said.
OK. I think I see what you mean by loaners and sellers (although, as I said, that terminology was not used).
For all practical purposes of understanding the situation, assume that any loan that a bank gives to 1 contractor goes to one other business. In reality, the loan goes to pay for employees, business expenses to multiple businesses, stays on hand for petty cash, etc. However, at the end of the day, the vast majority of the capital winds up back in the banks.
Even if 80% is re-deposited back into banks, you still have money multiplication, just not at the extreme end if 100% was re-deposited. See @sigmajin's example where some of the cash was kept on hand from various businesses.
For the purpose of instruction, aggregating totals of payments of a business into a single entity (when the practical mathematics are the same) is easier to explain the mathematics of an infinite geometric series.
Being paid to explain the scam of money creation, with the effect of making this information being wildly disseminated, we'll only ever see this on Steemit, and that' why the dumb stream media won't survive the Steem Stream Media!
Jesus-Christ. I was sure I had upvoted this article back then. Damn. Anyway I upvoted it now. It's an important article. I love it.
Oh okay I had. It's because it's the second payout! I hope you or other people will make post about the money creation scheme. It's so central to our lives. Thank you again!
"The reserve is not needed. Once the agreement between who prints the money and who makes them real utilizing them is established, the monetary function can be based on anything virtual. The issue is that if who owns the privilege of seignorage decides to put everybody in debt, and this debt can't be paid back with the same means we've got exponential slavery."
Cit. Giacinto Auriti vs. Bank of Italy 1992,
To be fair, the economists are correcting me in my understanding of economic theory.
However, the argument that money multiplication is a myth only applies to this application of an infinite geometric series in that loans are made from deposits.
OK. But money multiplication still occurs since banks can loan any amount they want to (assuming credit-worthiness of the borrower) and if they don't have the reserves, borrow the appropriate amount from a central bank to meet reserve requirements.
The total amount of money that is lent out is still larger than the total amount that was originally borrowed from the central bank. Hence, money multiplication is not a myth.
Though i accept it (since i agreed to it), i should have corrected your earlier definitition of the money multiplication effect.
The conventional use of the term, like in old econ text books, is what you describe in your post... the notion that a fractional reserve allows for the creation of money through loans in the infinite geometric pattern you describe. This is false.
Yes, you could say that banks create money through loans. It isnt strictly accurate, because its really the fed thats creating it, just like they create all money.. but yeah, its being created. Think of it this way. Berniesansders upvoted my reply earlier itt, and that upvote made me $800 bucks (which, btw, thanks bernie). I didnt get the 800 from bernie, though. He wasn't giving me his money. You could argue that bernie created that money by voting... and i suppose you'd be partially right (as right as you are about banks creating money through lending). But really what created that money was the steem "central bank", a thing i call the Vault thats modeled off the federal reserve and i don't think anyone else has really noticed yet. Someone else created the money -- Bernie just decided who to give it to. (in that analogy SP holders are like commercial banks and the vault is like the fed, which doesnt completely work but you get the point)
But thats a good thing... Money has to be created somehow. And if its just the government pumping money into the system, the only people who will have money is government cronies. If youre talking about the types of countries that one would generally describe as "free capitalist nations" like the US, UK, western europe etc, this proccess is what does most of the work re: increasing the money supply.
The alternative -- the other way to increase the money supply that places like the soviet block and military juntas and such is political or military crony-ism. It means that most people will never have a chance to get the new money thats being put into the system... because the government gives it to politicos, then it just stays with them (instead of being used to fund loans which fund jobs, etc etc)
An economy where the money supply is not growing, is a dysfunctional economy. I know the term "inflation" and "monetary inflation" gets thrown around a lot (i dont really get it, but i know it. All these 20-somthing blockchain computer programming hashtag using github posting millennials have the economic sensibilities of 19th century austro-hungarian viscounts), but the fact is that if you live in a country with a non-growing money supply, its probably in a mud hut and you have flies landing on your eyeball every couple minutes.
My main point of contention with the article was the strongly implied notion that the creation of money in this manner was that this sort of money creation was a "hack" -- an unintended vulnerability in the economic system that banks were exploiting for their own ends, by creating infinite amounts of money, and to the detriment of others. When, in fact, it is an intended design point without which quality of life in the US would be significantly lower than it is today.
I understand that the conventional use of money multiplication is the manner in which I described it in the OP. However, I would think that the common person would agree that any amount of money that is more than the amount initially borrowed from a central bank that is in circulation would necessarily be money that had been multiplied.
I could care two hoots if MM happens through deposits being lent out in the scheme initially described or if loans are made with rational business / banking decisions and then banks borrow from the central bank (or do overnite lending from others) to meet RR. To me, MM happened, regardless.
Japan has had a deflationary monetary supply since after WWII. I don't think they are all living in huts.
So population has increased in japan since the end of WWII... but the money supply has decreased. are they all just getting poorer? Or has their economy been so closely linked to the US that much of their corporate infrastructure uses dollars instead of yen, and holds them in US banks instead of japanese banks. (its the second thing)
Japan is an interesting exception to a general rule, and it comes mostly from close ties to the US and the US economy. In reality their money supply has grown... its just that most of that supply is in dollars. China is actually moving toward the same problem with bitcoin right now, though itll be a long time before it gets that bad.
That said, the BOJ has a NIRP going for like 3 years now.... they understand its a problem. THe guy who runs the BOJ would probably kill his mom to get the kind of geometric progression youre talking about.
NIRP means negative interest reserve policy. It means that if a japanese bank has a reserve higher than the required reserve ratio, the bank actually has to pay a fee (theyre charged negative interest on the extra) The purpose is to try to encourage japanese banks to act more aggressively in the lending market, rather than let their money sit in reserve. The problem is japanese corporations keep borrowing from US banks, because everyone there takes dollars, and US banks offer more competitive terms. ...
And I never meant that money creation in and of itself is a hack or a vulnerability of the current economic system. In fact, MM / or MC still happens regardless (as mentioned in a different reply). I just wanted to discuss some math and how I understood it to relate to economics (even if I was wrong in the example, the math holds).
Which reminds me, no one ever made a guess on this.
I would have accepted payday loan stores like the money store or loansharks. Partial credit for venture capitalists, since theyre investments, not really loans.
Point being that yes, there are people that will take their very own money out of their pocket or bank account and do what banks won't -- lend it to you directly. But they're taking way more risk and, as a result, they charge 40,000% apr and have terrible terms and possibly cave your head in with a crowbar if you miss a payment.
The balance sheet of the bank always has to balance - remember the bank lends to the same people it borrows from (as for every lender there is a borrower) so that's impossible. The more money the bank makes, the more the equity holders and deposit holders get. It's a circle that balances out to 0.
https://steemit.com/finance/@gleepower/the-definition-of-a-bank
I had intended to be the first steemian to use the word 'asymptotic'. Oh well.
@complexring Nice analysis. One thing to note about modern banking is that they don't use the money multiplier effect. I know it's taught in all the econ departments in all the schools and even Austrian economists I follow describe the money multiplier effect, but that's not how banks operate. They just create money ex-nihilo. Here is the Bank of England Quarterly Bulletin: Money Creation in the Modern Economy. Yes commercial banking is corrupt and a monopoly, but the money creation process banks use actually make sense. They just create loans from asset collateral (mainly real estate) and use these loans as monetary assets for commerce. It's actually the same as how Bitshares & Steem dollars work. Instead of real estate collateral, Bitshares uses BTS and Steem Dollars use Steem. I do agree banks are monopolistic, the Federal Reserve system is unnecessary and QE is harmful, but I've come to accept money creation via loans on assets as a good way to create monetary liquidity. Took me a decade of study on and off and banging my head against a wall trying to get to the bottom of the Federal Reserve and the money system. For most of that time I considered the whole system a conspiracy and a fraud especially after reading the legal theory of deposit contracts in Jesus Huerta de Soto's book Money, Bank Credit, and Economic Cycles ... I have since changed my perspective. Anyways the Federal Reserve and modern banking is really an esoteric subject. Intentionally so it seems.
@complexring From the title of this post I assumed that you indeed thought "the whole system a conspiracy and a fraud". How would you describe the banking system now that would conflict with that?
My understanding of it basically follows the 'Money as Debt' video below.
The dollar as debt instrument is a hard concept to understand at first
Well, certain countries don't use fractional reserve banking. The UK is one. You'll note that I gave a list of countries that don't have a reserve requirement. Only when a reserve requirement is needed, does the money multiplier effect come into play. Actually, with no reserve requirement, the money multiplier effect is infinite, which is effectively the same as creating money ex nihilo.
complexring, i love your name; and I've been impressed with your stuff; I added you to my green-list of good authors and articles, here. But I'm commenting to thank you for your perspective. I recently wrote about #wealth-distribution but with much less elegant math.
you just made me 800 steem and my post go viral, thankyou
I assure you that I did nothing of the sort.