Why Does A Sphere Roll So Smoothly?
Why Does A Sphere Roll So Smoothly?
This is part 2 of my “rolling” post. This time it is about rolling in 3D space.
Just like in the first part we can define rolling smoothly as the action of a shape translating a horizontally by rotating and stay the same horizontal height. Thus a sphere is a perfect example of such an object. You can get a sphere by spinning a circle like this:

But is there other objects that has a constant width such as a sphere? Well of course the answer is simple yes! There is a large amount of these shapes! Here is one derived from the Reuleaux triangle You can make this shape by spinning the Reuleaux triangle like we did with the circle, it will look something like this:
You can roll this object in any direction or position and it will roll just as smoothly as a sphere! You could also roll a cube by changing the surface area like we did with the square like this:

But what if you rotate a square like the circle, could such a shape roll?
Well this is something that I have personally pondered on when I saw how short this post was, I thought that there might be something be interesting to be unveiled, and the answer is yes there is!
Note that if you rotate a square like we did with the circle we get a cylinder! This is not an object of constant width, because you could try and roll it like this and not find a smooth roll:
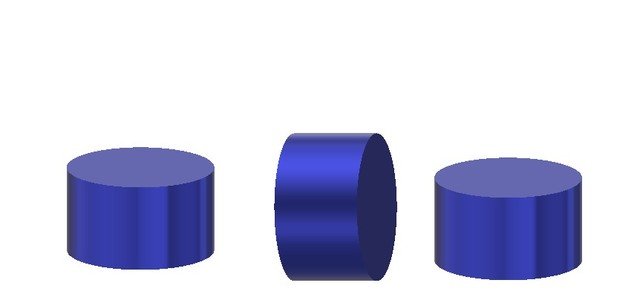
but if you roll it like this:
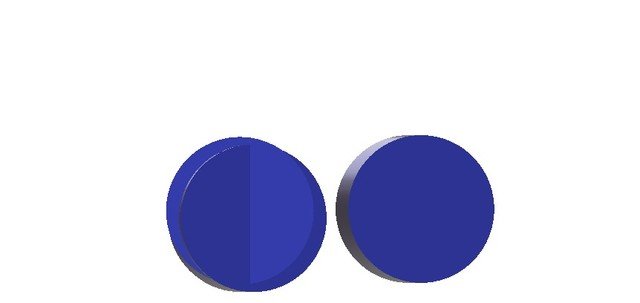
You will find that it is a smooth easy roll.
It would seem that all the shape non constant width shapes can form a non constant width three dimensional objects when spinned, but it has at least one position that one can roll smoothly where its two dimensional cousins does not have any position to roll smoothly!
I am excluding any triangle for it does not have an position that allows it to roll smoothly without rolling in circles!
As always thanks for reading!
what do you think? leave a comment down below. Do not forget to upvote, Resteem and follow my blog spot!
check out more posts Here
