Buoyant Force- The fluid force and it's impact on substance to substance
Some things floats on liquid say water, some get sinks and some of them get partially submerged.Now if I ask the reason behind it you will probably say well it depends on the weight of the object.Right? Okay you might know the answer of it but in this post I am going to describe the reason behind it thoroughly. I mean as good as I can try :)
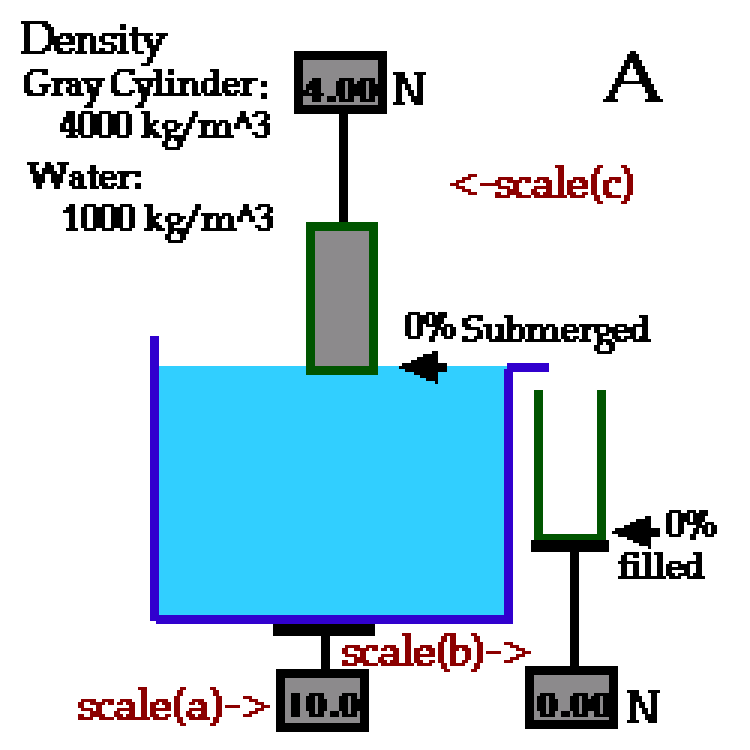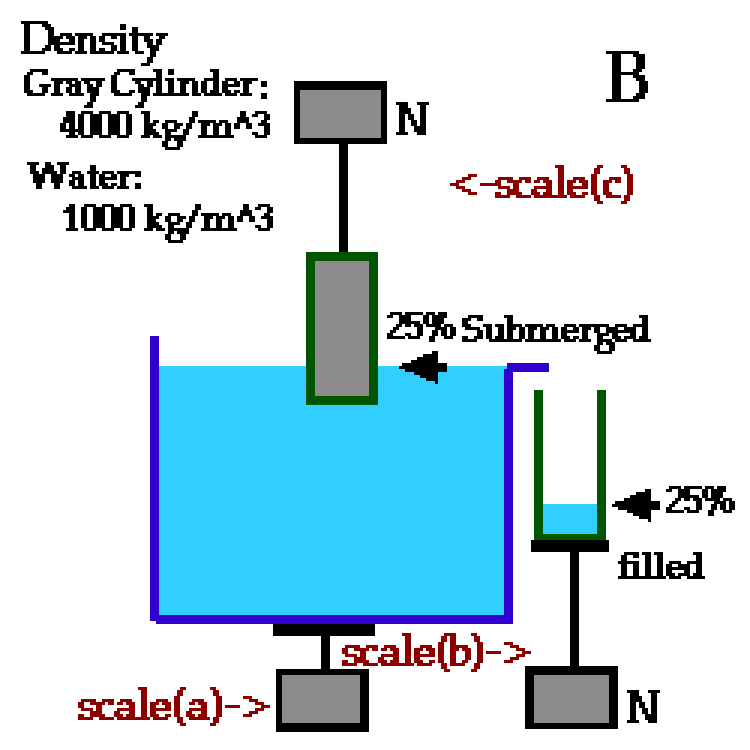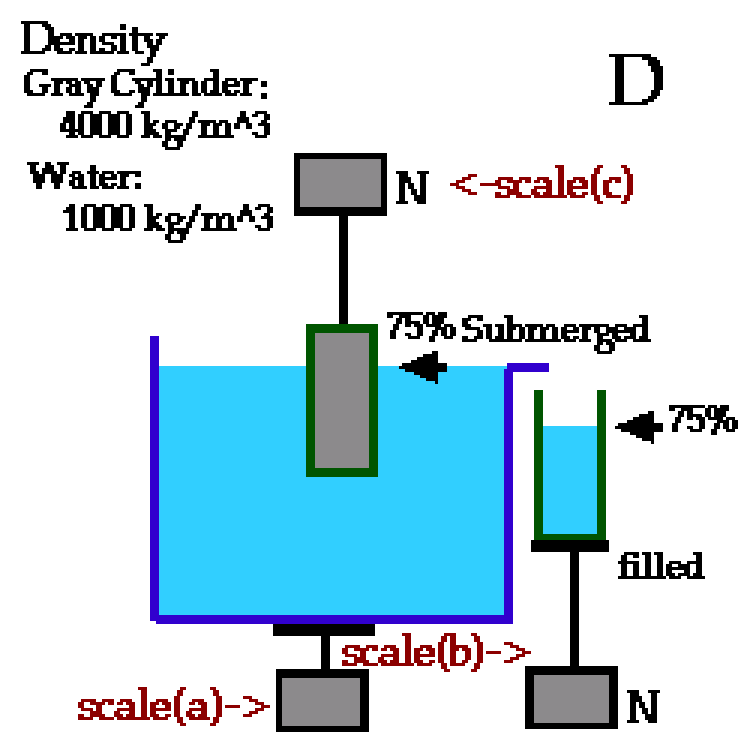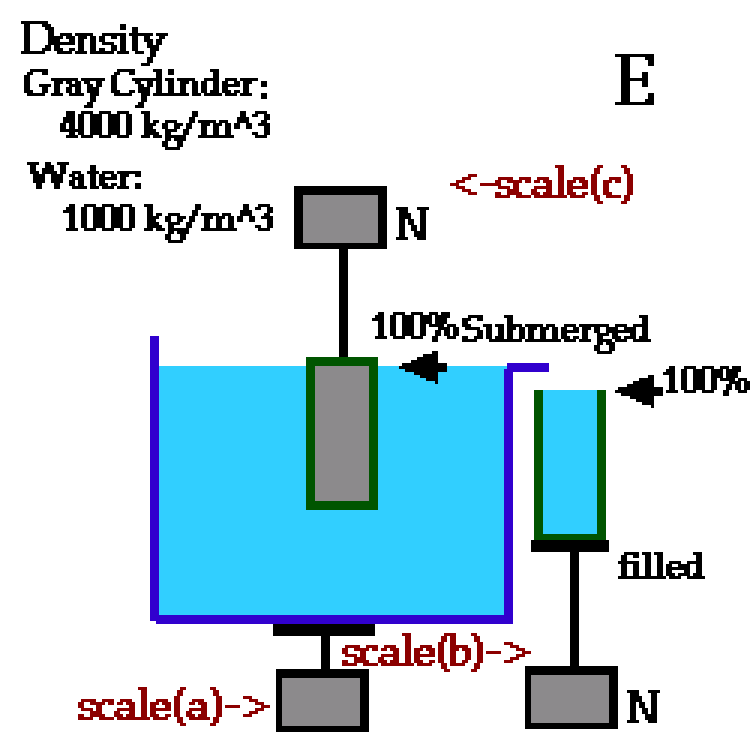
Around 250 BC the great Archemedes stated the reason behind it.According to his theory,
" When a body is partially or wholly submerged into a fluid at rest, the fluid exerts an upward force equal to the weight of the displaced fluid"
So basically fluid apply an upward force or thrust onto the object when it is dipped in it. This is the main point we have to focus on. To do so let me illustrate this with an example.
Say we have a cubical block of side a which is to be submerge on the fluid of density ρ which is at rest. Now when we submerge the block in the liquid it will experience two forces on its top and bottom faces. Since the fluid that we are taking is at rest so there are no horizontal forces to be include. There will be two force acting on the block, one on the top surface acting downward and the other will act on the bottom surface acting upward. Let us say F1 and P1are the force and pressure exerted on the top surface respectively. Similarly let's say F2and P2 are the force and pressure exerted on the bottom surface resp.
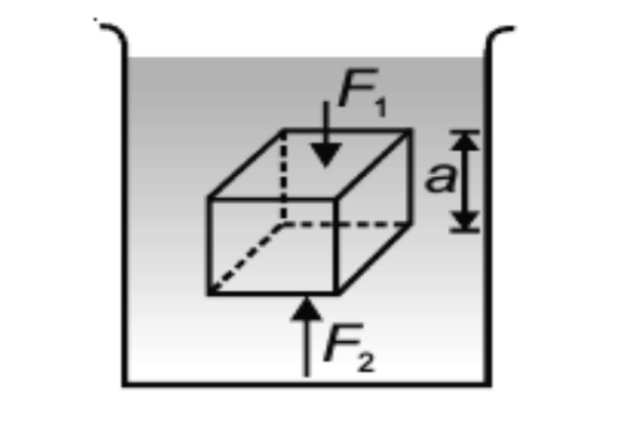
F1 = P1a2
F2 = P2a2
P2 = P1 + ρga
i.e P2 > P1
=> F2 = (P1+ρga)a2
=> F2 = P1a2+ρga3
=>F2 = F1+ρga3
So it is clear that F2 is greater than F1 by an amount of ρga3 and this difference in the two forces provides the resultant upward force (F2−F1) which is called the buoyant force or the upthrust(U)
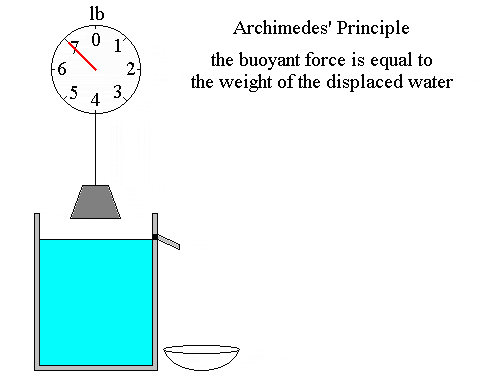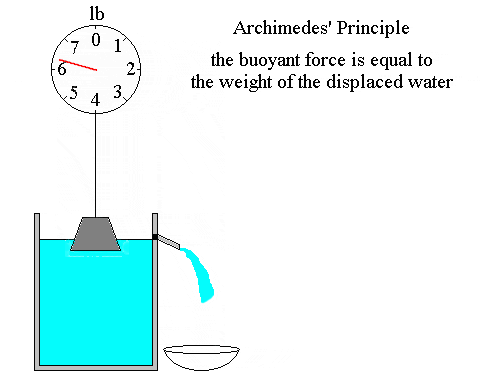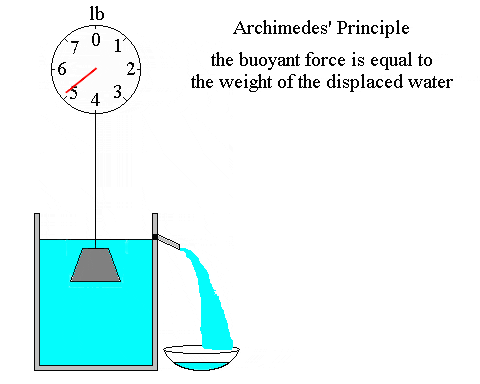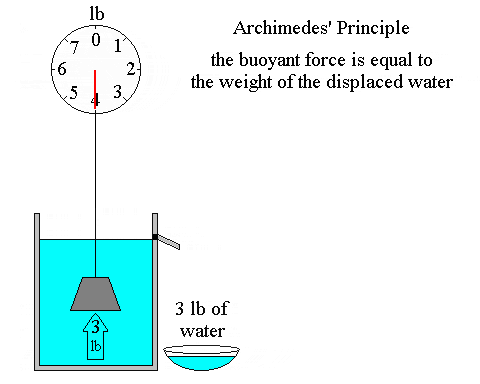
Therefore U=ρ×g×a3
Mass/Volume = Density
=>Mass = Density×Volume
=>M = ρa3
=>M×g = g×ρ×a3
=> W = U
Thus the upward force or the upthrust on the block is equal to the weight of the displaced fluid.The result is true for the body of any shape and for partially as well as fully immersed bodies.So the law can also be stated as,
The loss of weight of a body submerged in a fluid is equal to the weight of the fluid displaced.'
Now let us consider the density of the block is σ Now it's weight can be given as W= σga3.Since the weight acts downward and the upthrust U acts upwards. Therefore the apparent weight of the block when it is fully immersed in the fluid will be,
W' = W−U
= σga3−ρga3
= σga3(1−ρ/σ)
W' = W(1−ρ/σ)
Some cases:
1. When ρ=σ
W' = 0
i.e. upthrust U balances the weight of the block. The block floats completely submerged just below the surface of the fluid.
2. When ρ > σ
U > W
i.e. the upthrust U is greater than the weight of the block. This cause the block to be partially immersed in the fluid.
3. When ρ < σ
U < W
i.e. the upthrust U is not sufficient enough to balance the weight of the block and hence the block sinks.
Sources:
1.Acott, Chris (1999). "The diving "Law-ers": A brief resume of their lives."South Pacific Underwater Medicine Society journal.
2."Floater clustering in a standing wave: Capillarity effects drive hydrophilic or hydrophobic particles to congregate at specific points on a wave"
3."Archimedes’s principle gets updated". R. Mark Wilson, Physics Today 65(9), 15 (2012);
4."The works of Archimedes". p. 257. Retrieved 11 March 2010. Any solid lighter than a fluid will, if placed in the fluid, be so far immersed that the weight of the solid will be equal to the weight of the fluid displaced.
Image credit:
1-wps.prenhall.com
2-Phy book by www.aakash.ac.in
3-weclipart.com
If I missed anything important regarding the topic feel free to drop your point below in the comments section.All are welcome :)

@minnowpond1 has voted on behalf of @minnowpond. If you would like to recieve upvotes from minnowponds team on all your posts, simply FOLLOW @minnowpond.