A 'new' ‘Einstein’ equation suggests wormholes hold key to quantum gravity
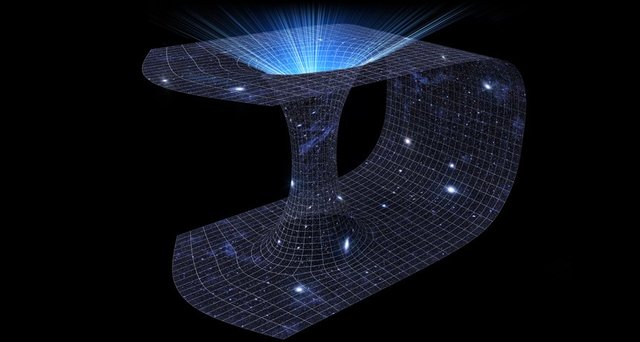
There’s a new equation floating around the world of physics these days that would make Einstein proud.
It’s pretty easy to remember: ER=EPR.
You might suspect that to make this equation work, P must be equal to 1. But the symbols in this equation stand not for numbers, but for names. E, you probably guessed, stands for Einstein. R and P are initials — for collaborators on two of Einstein’s most intriguing papers. Combined in this equation, these letters express a possible path to reconciling Einstein’s general relativity with quantum mechanics.
Quantum mechanics and general relativity are both spectacularly successful theories. Both predict bizarre phenomena that defy traditional conceptions of reality. Yet when put to the test, nature always complies with each theory’s requirements. Since both theories describe nature so well, it’s hard to explain why they’ve resisted all efforts to mathematically merge them. Somehow, everybody believes, they must fit together in the end. But so far nature has kept the form of their connection a secret.
ER=EPR, however, suggests that key to their connection can be found in the spacetime tunnels known as wormholes. These tunnels, implied by Einstein’s general relativity, would be like subspace shortcuts physically linking distant locations. It seems that such tunnels may be the alter ego of the mysterious link between subatomic particles known as quantum entanglement.
For the last 90 years or so, physicists have pursued two main quantum issues separately: one, how to interpret the quantum math to make sense of its weirdness (such as entanglement), and two, how to marry quantum mechanics to gravity. It turns out, if ER=EPR is right, that both questions have the same answer: Quantum weirdness can be understood only if you understand its connection to gravity. Wormholes may forge that link.
Read the original article here
For those who are read up on quantum theory and general relativity and want to go a little further into understanding this, Leonard susskind has done a lecture on the topic.