Basics of Rocket Propulsion - Part 1

Image Source

The Tsiolkovsky's Rocket Equation is V_t = v_e . log (M_o/M_t)

Here, V_t = velocity of the rocket at time t after its launch at t=0. This is just for simplicity, actually V is more accurately δV, the change in velocity. And δV = final velocity (V) – initial velocity (U), but since our rocket is being launched from earth surface and is quite stable (ignoring earth’s rotation) when it is launched, initial velocity can be considered zero which gives δV = V.

v_e is the rocket's exhaust velocity,
M_o is the initial mass of the rocket, before it was launched, without any fuel expelled out of it, or simply put, at t=0.
and M_t mass of the rocket at time t.

We see that in our equation, M_o is a constant. Also, since we want don't want to burn any fuel if we can't make the most out of it, v_e is always kept constant. We may use more or less fuel at different times, but we always push it out at the highest possible speed. M_t, changes as fuel is burnt and utilized, and this is the only variable in the equation which changes our rocket's velocity with time.

In his paper, Tsiolkovsky first derived the formula for escape velocity for an object. For earth's surface, this velocity was found to be 11.2 km/s, ignoring the atmospheric pressure, and friction caused by contact with air. This means if we ignore other factors that slow down the speed, and somehow launch a spacecraft from earth's surface at a speed of 11.2 km/s, we might get it in space, out of earth's gravitational field, without applying any other force on it. We have a story behind this concept.

When people first got the idea of escape velocity, they thought that a better option was to fire something in space, like a projectile. They imagined shooting a bullet up in the air with the speed of at least 11.2 km/s. And many tried to achieve this, obviously with no success, or we would have entirely different rocket launchers. Slowly, people gave up, and eventually turned to rockets as a means of space travel, an idea proposed by Tsiolkovsky in the very same paper. He also suggested using liquid hydrogen and liquid oxygen for propellant. So you see, a rocket is a vehicle. It uses fuel for movement, it's not a projectile. This idea has now evolved for space travel. These days, we have the solar and plasma sail technology which do not consume any fuel in space travel, but rather harvest energy that is readily available in space.

Now, back to our rocket equation, we find that the velocity of our rocket after its launch depends entirely on the initial and current mass of the rocket, and the exhaust velocity or the velocity with which the propellant escapes the rocket. The higher the speed with which the propellant is pushed backwards, the faster the rocket moves forward - which is due to conservation of momentum.

In the mass ratio M_o/M_t, which can be simply written as R, M_o is a constant since it is just the initial mass of the rocket along with all the fuel it had, and M_t changes as fuel burns. This was a tricky part of the equation. Let’s clarify a few things about it.

A mass ratio of say 5, means M_o/M_t = 5. At a time when all the fuel is utilized, this ratio is simply initial mass (vehicle mass + fuel mass) to vehicle mass, this is the highest value of mass ratio during the rocket’s journey. This means, the vehicle mass is one part and the propellant mass is 4 parts out of the initial rocket mass. Or simply put, 80% of the rocket’s initial mass is the propellant. Similarly, mass ratio of 10 or 20 means, fuel is 90% or 95% of the initial rocket mass. Also, you see, the rocket velocity varies as the log of this ratio R, which is always more than one, since M_o > M_t any time after the rocket is launched (t ≠ 0). And now we plot a graph between V and log (M_o/M_t) and different values of v_e. It's just a rough image by me, edited it a bit...

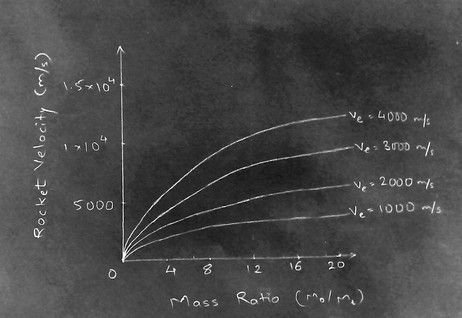
The problem here is, We need a high enough ratio of M_o / M_t, and high enough exhaust velocity v_e that would lead to the escape velocity of 11.2 km/s (in graph, a bit more than 10⁴ m/s) , if we are planning to launch a rocket directly from earth's surface, and get it beyond the earth's gravitational pull. We need propellant with the least mass, and that's why liquid hydrogen and liquid oxygen were proposed. Tsiolkovsky also suggested solid, metallic hydrogen to be used, which is something we haven't yet really figured out how to create, but we are on our way. We are trying...

But the better solution to this problem is using multistage propulsion. In the first stage, we simply carry the rocket in the lower earth orbit. And in second stage, from the lower earth orbit, we later move it out of the earth’s gravitational pull, which is more feasible. Also, the escape velocity is much less above the earth’s surface because it varies inversely with square root of the distance of object from earth’s center. (v_e α 1/√R)

If you look at the graph we plotted for velocity of rocket versus the mass ratio, you can see that after some point, it tends to become constant. This points us to the fact that, after some point, adding more fuel to the rocket doesn't make much difference, this is explained above already. For mass ratio of 5, 10, 20, and 40, fuel mass is 80%, 90%, 95% and 97.5% of the initial mass of the rocket.

21 years after publishing this paper, in 1924, Tsiolkovsky published one more paper in which he proposed multistage rockets, the ones we currently use. That's it for today, we will continue our story later.

See ya'll soon...Take care.

References:

1. https://en.wikipedia.org/wiki/Tsiolkovsky_rocket_equation
2. https://en.wikipedia.org/wiki/Hero_of_Alexandria
3. https://en.wikipedia.org/wiki/Space_gun
4. https://en.wikipedia.org/wiki/Single-stage-to-orbit
5. And my lecture notes